Electrorheology denotes the control of a material’s flow properties (rheology) through the application of an electric field. ER fluid was discovered sixty years ago. In the early days the ER fluids, generally consisting of solid particles suspended in an electrically insulating oil, exhibited only a limited range of viscosity change under an electric field, typically in the range of 1-3 kV/mm. The study of ER fluid was revived in the 1980’s, propelled by the envisioned potential applications, as well as the successful fabrication of new ER solid particles that, when suspended in a suitable fluid, can “solidify” under an electric field, with the strength of the high-field solid state characterized by a yield stress (breaking stress under shear). However, further progress was hindered by the barrier of low yield stress (typically in the range of a few kPa).
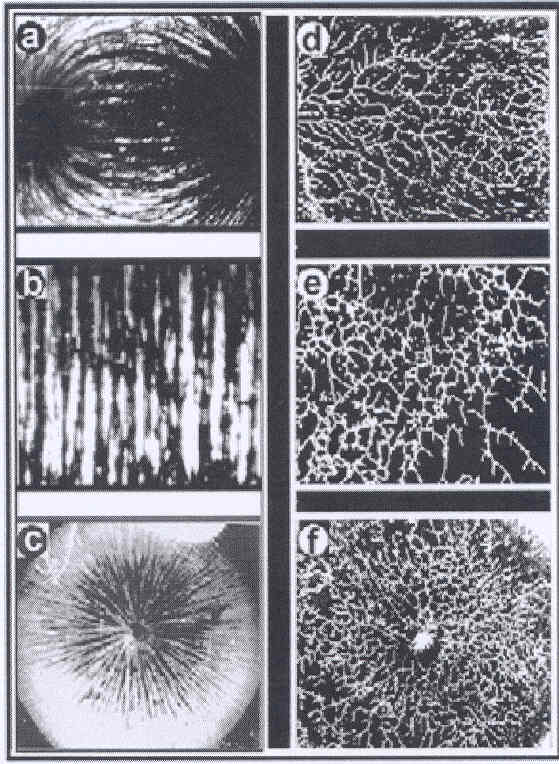
Starting in 1994, we have adapted the mathematics of composites, in particular the Bergman-Milton representation of effective dielectric constant, to the study of ER mechanism(s). The questions we aimed to answer were: (1) the role of conductivity in the ER effect, (2) the role multipole interaction, (3) the ground state microstructure of the high-field state and, most importantly, (4) the upper bounds in the yield stress and shear modulus of the high field solid state. Finding the answer to (4) led to the suggestion of the coating geometry for the ER solid particles which can optimize the ER effect, but at the same time also pointed out the limitation of the ER mechanism based on induced polarization. The subsequent study of adding controlled amount of water to the ER fluid pointed to the intriguing possibility of using molecular dipoles as the new “agent” for enhancing the ER effect. Working along this direction, experimentalist Weijia Wen was able to synthesize urea-coated nanoparticles of barium titanyl oxalate which exhibited yield stress in excess of 100 kPa, breaking the yield stress upper bound and pointing to a new paradigm in ER effect in which the molecular dipoles can be harnessed to advantage in controllable, reversible liquid-solid transitions with a time constant on the order of 1 msec. We propose the model of aligned surface dipole layers in the contact area of the coated nanoparticles to explain the observed giant ER effect, with the electric-field induced dissociation (the Poole-Frenkel effect) of the molecular dipoles accounting for the observed ionic conductivity. Quantitative agreement between theory and experiment was obtained. There are still many intriguing questions yet to be answered regarding the GER effect. While GER represents a big step towards realizing the application potentials of ER fluids, there are still some problems to be solved before ER fluids can become a commercial reality.
Key publications:
- “Frequency Dependent Electrorheological Properties: Origin and Bounds“, H. R. Ma, W. J. Wen, W. Y. Tam, and P. Sheng, Phys. Rev. Lett. 77, 2499 (1996).
- “Frequency and Water Content Dependencies of Electrorheological Properties“, W. J. Wen, H. R. Ma, W. Y. Tam and P. Sheng, Phys. Rev. E 55, R1294 (1997).
- “New Electrorheological Fluid: Theory and Experiment“, W. Y. Tam, G. H. Yi, W. J. Wen, H. R. Ma, M. M. T. Loy, and P. Sheng, Phys. Rev. Lett. 78, 2987 (1997).
- “Ground States of Magnetorheological Fluids“, L. Zhou, W. Wen and Ping Sheng, Phys. Rev. Lett. 81, 1509 (1998).
- “Field Induced Structural Transition in Mesocrystallites“, W. J. Wen, N. Wang, H. R. Ma, Z. F. Lin, W. Y. Tam, C. T. Chan, and P. Sheng, Phys. Rev. Lett. 82, 4248 (1999).
- “Robust Photonic Band Gap from Tunable Scatterers“, W. Y. Zhang, X. Y. Lei, Z. L. Wang, D. G. Zheng, W. Y. Tam, C. T. Chan, and Ping Sheng, Phys. Rev. Lett. 84, 2853 (2000).
- “The Giant Electrorheological Effect in Suspensions of Nanoparticles“,W. J. Wen, X. X. Huang, S. H. Yang, K Q. Lu and P. Sheng, Nature Materials 2, 727 (2003).
- “Dielectric Electrorheological Fluids: Theory and Experiment“, H. R. Ma, W. J. Wen, W. Y. Tam and P. Sheng, Adv. Phys. 52, 343 (2003).